Odd, isn’t it?

This past year I’ve been thinking a lot about Math and how students learn about numbers. Our district Math coach has been really pushing us to check for understanding by giving students lots of tasks that require them to use what they know about numbers in new ways. And, of course, every step along the way we ask the question “How do you know?” In the past, when thinking about teaching the difference between odd and even numbers, I think I would have looked at it as a memorization task. Even numbers are the multiples of 2; odd numbers are the other ones. Easy. But our latest Math Module keeps asking that how do you know question so I needed to change my thinking.

Here’s how we started. I read the book Missing Mittens by everyone’s favorite mathematical storyteller Stuart J Murphy. It’s a simple story, really intended for younger students than my second graders, but it made for a quick and fun read. It also helped solidify the concept of pairs, which is an important vocabulary word for the unit. (And, one star student connected it to the work we’ve been doing on homophones – pair and pear – gotta love it. We talked about the multiple meanings of the words odd and even later.)

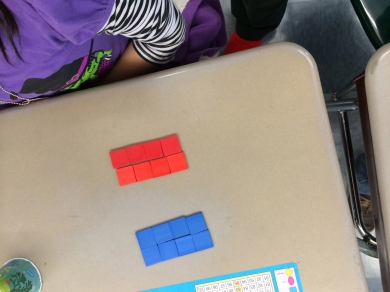
The book nicely illustrates each number of mittens in a box labeled odd or even. So, after reading the book, we looked at the day’s objective: We will be able to show an even number with tiles and tell how we know the number is even. All the students got a cup of tiles and took out an even number. They worked with a partner to arrange the tiles in a way that showed the number was even. A few teams needed a quick prompt of “But, how do I know this is an even number?” but everyone quickly arranged the tiles.

When we were sharing arrangements, I wrote down the language they used to prove their collections showed an even number and posted the sentences on our Math board. I’m trying to get better at offering these language supports that we can refer to throughout the unit to help students talk and write about their thinking. It’s not natural yet for me or for them, but we’re all getting better. I went back to the sentence strips later to highlight the vocabulary for the unit and now I’ll add these pictures of how they showed their work so my ELLs have the visual support they need.

The next day, our goal was to find some rules about adding and subtracting odd and even numbers. We tested each one to determine the rules. The kids had a blast making predictions, letting out big groans when their predictions were disconfirmed, giving high fives when they were proven right.

These two days are leading us into work with arrays to show repeated addition and help prepare them for multiplication work (which most of them will do in 3^rd grade). Now that I have done all the work of thinking about the real understanding students have to have about numbers, I think this unit on arrays will go a lot more smoothly and be a lot more productive. My students are beginning to really understand numbers in a way that will allow them to be flexible in their thinking rather than follow meaningless rules.

I can’t wait to dive in on Monday.

Counting

So, the idea was to see if my second graders could apply what we have learned about place value and groups of ten to counting a large collection. The results were interesting.

I gave each pair of students a bin full of math materials. Each bin had over a hundred pieces in it and when they heard the task there were a lot of surprised gasps. Count all this? It sounded impossible, but they were game. The instructions I gave were these:

Talk to your partner about the best way to count everything in your box and then count everything in your box.

Simple.

As they got started, I walked around and observed. When I saw one of my usually strong partners begin counting by ones, moving each block from one pile to the next, I let him get to about 30 and then I interrupted him, trying to get him to lose count. Success. One bright student remembered counting by twos from the first grade so immediately convinced his partner to stack the blocks in pairs. When I stopped in to hear their strategy I added “Oh, did I forget to tell you that you can only use your two desks? I hope you don’t run out of room!” He looked questioningly at his partner with a “what now?” sort of face.

I let the struggle go on for a little while and when I noticed a few partners had worked out that they could stack the objects in groups of ten, I stopped everyone and asked a few teams to share their counting strategies. Then, I asked them to talk to their partners about which strategy they thought would be most efficient. (We’ve been working on that vocabulary word.) After listening to each other’s ideas, almost all teams started using the groups of 10 strategy. A quick conference with the one rebellious partnership revealed some miscommunication and partnership discord. Easily remedied.

Here’s some of the work:

Before clean up time, all the partners had successfully counted their boxes. Some had 120 items, a few had over 300. I can’t wait to see their faces when I tell them I forgot to write down their counts so we need to take inventory again. After all, practice makes proficient.

Math woes

This weekend I corrected the first Math test of the school year and I feel like a failure. There are certain questions that nearly everyone got wrong. Some I know I did not spend enough time on with the class. We should have practiced more; I should have taken the time to explain things in different ways; I should have made sure to use the same language and structure as the test question; I should have . . .  well, should have, but didn’t. And now their tests scores are set and there’s no going back.

So, of course, the first thing I did was make plans to go back. I’m not having students retake the test, nor will I doctor scores, but we have a little wiggle room before I need to start the next unit so I’m spending some time re-teaching. If this is important to know, then it’s important to try again.

We also have the advantage of being able to form our own groups for the district mandated Math Intervention. While I am not a fan of this intervention model, I do appreciate that we are given some control over it. So, I am going over the formal test and some less formal assessments to see which standard each student needs to work on. If they don’t have the base ten system under their belts, then they are not ready to develop efficient strategies for working with larger numbers. If they can’t fluently work with numbers under 20, then let’s leave the base ten blocks in the tub and begin with some basic number sense and mental addition strategies.  I’ve started plugging my students into a table to help sort them into groups. The other 4 teachers on my second grade team will do the same. Then we’ll figure out the logistics of getting them into groups.

And, beyond those groups, I’ll spend a little of my Core Math time making the connection between this work with addition and subtraction and the upcoming unit on measurement.

Math is NOT my favorite subject so spending so much mental and creative effort here is not easy for me. However, Math is the favorite of many of my students and I owe it to them to help them reach grade level standards, and beyond. So, here I go.